

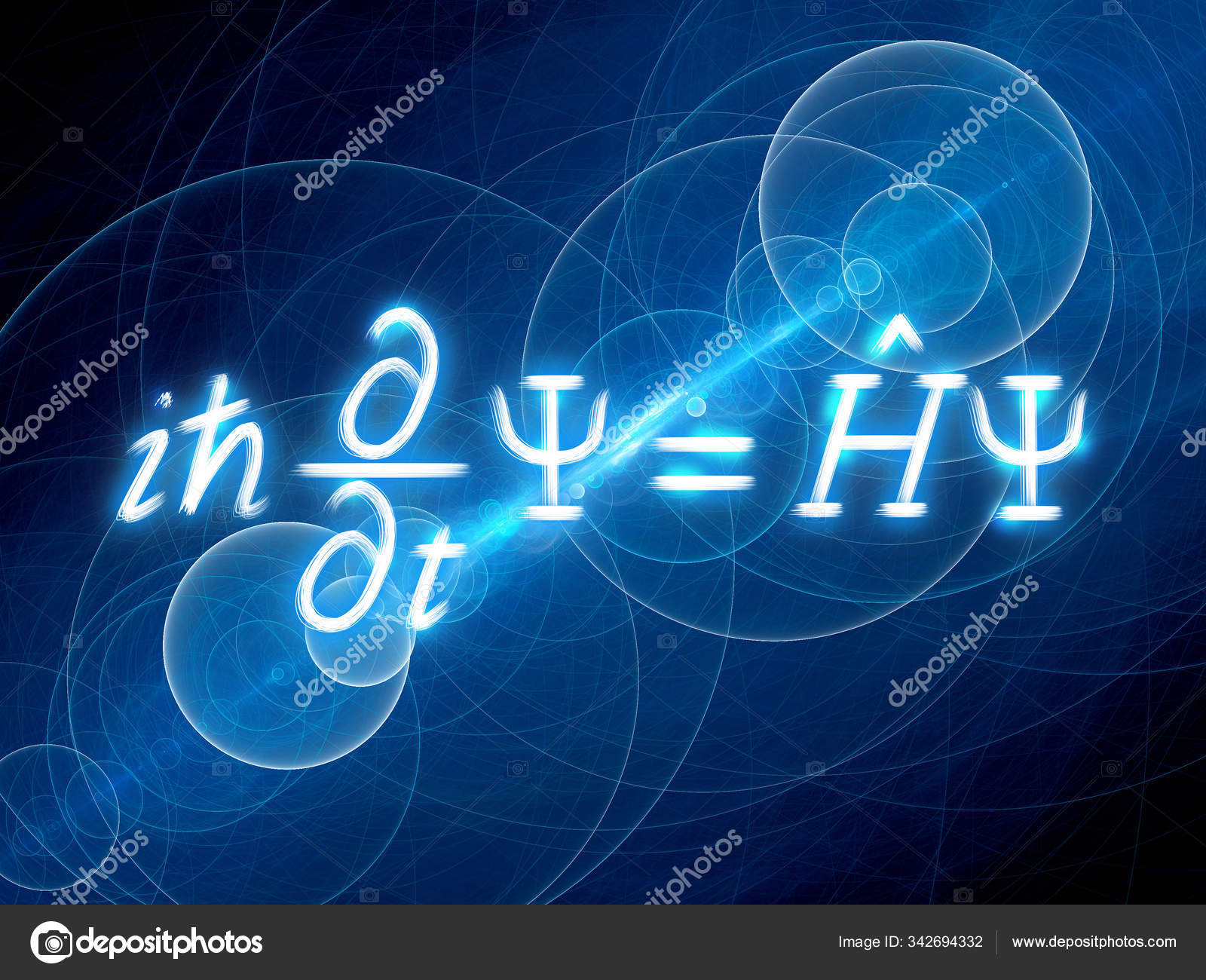
SCHRODINGER PICTURE PDF
Size | PPT Slide | PDF 2 Behavior of expectation values as a function of time
The expectation value of the dimensionless position operator as a function of time. An example can be found in, where the effect of an external sinusoidal field is considered.įig. The Morse coherent states can be prepared by an appropriate electromagnetic pulse that drives the vibrational state of the molecule starting from the ground state into an approximate coherent state. 1 indicates that initial displacements, x 0, having the order of magnitude of unity will not lead to “ small oscillations”. The absolute square of the wave functions |〈 x|0, 0〉| 2 and |〈 x|0.5, 0〉| 2 is depicted in Fig. That is, this molecule has 55 bound states, and we found that a basis of dimension N + 1 = 150 is sufficiently large to handle the problem. In our calculation we have chosen the NO molecule as our model, where m = 7.46 a.u., D = 6.497 eV and α = 27.68 nm -1, yielding s = 54.54. These plots correspond to the case of the NO molecule, where s = 54.54.
SCHRODINGER PICTURE MOVIE
The time evolution of the latter initial wave function is shown in the attached movie file. The absolute square of the wave functions corresponding to the Morse coherent states | x 0,0〉, with x 0 = 0.0 (ground state) and x 0 = 0.5. ( 4) is in one to one correspondence with the expectation valuesįig. ( 4) are “single mode” coherent states in contrast to those of, where the dynamics of two-mode coherent states were investigated for various symmetry groups, including SU(1,1), which is in a close relation to the relevant symmetry group of the Morse potential. The details of the construction of our basis can be found in. 〉, n > follow densely each other, approximating satisfactorily the continuous energy spectrum. The first +1 elements of the basis n = 0 N are the bound states, and the continuous part of the spectrum is represented by a set of orthonormal states which give zero overlap with the bound states. Where 2F 1 is the hypergeometric function of the variable 1 - β. Our description of molecular vibrations is based on the Morse Hamiltonian, that can be written in the following dimensionless form Revivals and the formation of Schrödinger-cat states demonstrating the genuinely quantum nature of the problem will be visualized here with showing both the wave packet motion and the time evolution of the corresponding Wigner function.

We are going to investigate here the role of anharmonicity in the realistic case of a vibrating diatomic molecule having a finite number of bound eigenstates together with a dissociation continuum. Peculiar quantum effects of wave packet motion in anharmonic potentials have been predicted in several model systems. ©2002 Optical Society of America 1 Introduction Our numerical calculations are based on a novel, essentially algebraic treatment of the Morse potential. We analyze the degree of nonclassicality as the function of the expectation value of the position in the initial state. These nonclassical states are coherent superpositions of two localized states corresponding to two different positions of the center of mass. We present animated wave functions and Wigner functions of the system exhibiting spontaneous formation of Schrödinger-cat states at certain stages of the time evolution. We investigate the time evolution of Morse coherent states in the potential of the NO molecule.

Note: Author names will be searched in the keywords field, also, but that may find papers where the person is mentioned, rather than papers they authored.Use a comma to separate multiple people: J Smith, RL Jones, Macarthur.Use these formats for best results: Smith or J Smith.For best results, use the separate Authors field to search for author names.Use quotation marks " " around specific phrases where you want the entire phrase only.Question mark (?) - Example: "gr?y" retrieves documents containing "grey" or "gray".Asterisk ( * ) - Example: "elect*" retrieves documents containing "electron," "electronic," and "electricity".Improve efficiency in your search by using wildcards.Example: (photons AND downconversion) - pump.Example: (diode OR solid-state) AND laser.Note the Boolean sign must be in upper-case. Separate search groups with parentheses and Booleans.Keep it simple - don't use too many different parameters.


 0 kommentar(er)
0 kommentar(er)
